23
Novembre
ore
12.00-14.00
Aula
Consiglio
|
Irene Giardina
Birds,
spins and entropy: a theoretical physics
approach to collective animal behaviour
Flocking
is a paradigmatic example of self-organized collective behaviour, where
collective ordering emerges from the mutual interactions
between individuals.  In this respect, it shares striking
similarities with collective phenomena in inanimate systems that have
long been studied by the physics community. Still, biological systems
are more complicated than physical ones and it is not evident whether
they can be described by the same kind of general laws so well
understood in physics. Experimental findings often go beyond simple
expectations, making this field even more fascinating.
In
this talk I will discuss our attempts to study collective animal
behaviour using a physicist's perspective. I will show how we
used concepts and methods from statistical physics to make sense of
experimental data of large flocks of birds. In particular, I will focus
on the velocity correlation functions, that well capture the balance
between consensus and independence among the large number of
individuals in the group. We measured these correlations in real flocks
of starlings and found that they exhibit a non-trivial scale-free
behavior, indicating a surprisingly large degree of coordination and
collective response.
I
will describe how we can use these correlations to systematically
address the inverse problem, extract from the experimental measurements
information on the underlying microscopic interactions, and build
minimal (maximum entropy) models directly from the data.
In this respect, it shares striking
similarities with collective phenomena in inanimate systems that have
long been studied by the physics community. Still, biological systems
are more complicated than physical ones and it is not evident whether
they can be described by the same kind of general laws so well
understood in physics. Experimental findings often go beyond simple
expectations, making this field even more fascinating.
In
this talk I will discuss our attempts to study collective animal
behaviour using a physicist's perspective. I will show how we
used concepts and methods from statistical physics to make sense of
experimental data of large flocks of birds. In particular, I will focus
on the velocity correlation functions, that well capture the balance
between consensus and independence among the large number of
individuals in the group. We measured these correlations in real flocks
of starlings and found that they exhibit a non-trivial scale-free
behavior, indicating a surprisingly large degree of coordination and
collective response.
I
will describe how we can use these correlations to systematically
address the inverse problem, extract from the experimental measurements
information on the underlying microscopic interactions, and build
minimal (maximum entropy) models directly from the data.
|
26
Ottobre
ore
12.00-14.00
Aula
Consiglio
|
Adriano
Barra
Collective phenomena in immune systems

The
immune system defends us from pathogens. But what is an antigen? Does
each lymphocyte really know the complete ensemble
of all the
molecules of our body? Is there, instead, a systemic cooperation among
these cells resembling neurons in the brain? Is the immune
system able to "think"? And, even more interesting, what is self? Is
there a sharp separation between self and not-self?
From the pioneering papers by Jerne, Varela and
Couthino, the idea of a network of B-clones interacting by exchanging
antibodies was formulated as an hypothetical way for explaining
tolerance toward self proteins by mature peripheral B-cells (beyond
clonal deletion and receptor editing during the ontogenesis). However,
despite anti-antibodies are commonly encountered, evidence in
favor of an extensive network is still lacking and even worse, always
sharper experiments (e.g. the works by Goodnow) highlighted strong
evidence of a phenomenon called "anergy": the latter shifts the
responsibility of this lacking of attack by B-cells self-directed to a
lacking of signalling by helpers cells (the second key needed for
B-cell activation beyond the primary antigenic target, self or
not-self): In a nutshell, B-cells need two activation signals, the
former being the target (antigen) the latter being a "consensus" by the
helpers. Helpers do not allow this consensus and the resulting B-cell
with only one signal undergoes into a regime of "anergy". But how can
helpers recognize those B-cells self-directed? The problem seems only
shifted... Coupled to a large introduction to theoretical
immunology and its formalization through statistical mechanics, we try
to revise this discussion within a thermodynamical framework by using
the tools of disordered systems.
|
1
Giugno
ore
12.00-14.00
Aula
Consiglio
|
Giovanni Alberti
Variational problems on the equilibrium (and quasistatic evolution) of
drops
The
classical (that
is, geometric) model of capillarity accounts for many of the
equilibrium shapes we commonly observe in liquid drops. In fact, even
more complicated phenomena such as superhydrophobicity and contact
angle hysteresis can be explained using simple variants of the basic
model. In this lecture I will start from the classical theory to
arrive, if time permits, to a recent work in collaboration with Antonio
De Simone on the quasistatic evolution of drops.
|
4
Maggio
ore
12.00-14.00
Aula
Consiglio
|
David Quéré

On the shapes of water
As
we learnt from Young and Laplace, the cohesion of fluids makes them
choose specific shapes, in particular spheres at a small scale. We
discuss several ways to maintain this ideal shape on a solid, which
leads to unique dynamical situations: water pearls do not
stick, they
run
easily and they bounce - a little bit as if they were solid marbles.
(But they are not. And the liquid nature of these pearls has interesting
consequences on the dynamical shapes they adopt, for example.) The high
mobility of liquid pearls implies that tiny forces are sufficient to
move them, and we plan to present recent achievements where some
asymmetric patterns at a solid surface permit the self-propulsion of
the liquid. We would also like to discuss the effects of various fields
to control these elusive drops.
|
30
Marzo
ore
12.00-14.00
Aula
Consiglio
|
Lorenzo Giacomelli
Mathematical models of wetting phenomena
 Wetting
phenomena at "small" scales (a water drop on a glass, the precorneal
tear-film) may be described by quite a few different mathematical
models: diffuse interface ones, sharp ones such as the Navier-Stokes
equations, and reduced ones such as the lubrication and the
quasi-static approximations. Furthermore, they open up fundamental
questions whose answer is yet debated, such as the description of the
interface (if any) which separates "dry" from "wet" regions. Which
model and which answer are most appropriate is likely to depend on the
physics of the specific phenomena, and I will provide introductory
information for most of them. However, all of these models are grounded
on a basic and unifying physical mechanism: the balance of capillary
and frictional (e.g. viscous) forces. Enlightening this principle will
hopefully help understanding and enjoying the subsequent lectures
within this series. Wetting
phenomena at "small" scales (a water drop on a glass, the precorneal
tear-film) may be described by quite a few different mathematical
models: diffuse interface ones, sharp ones such as the Navier-Stokes
equations, and reduced ones such as the lubrication and the
quasi-static approximations. Furthermore, they open up fundamental
questions whose answer is yet debated, such as the description of the
interface (if any) which separates "dry" from "wet" regions. Which
model and which answer are most appropriate is likely to depend on the
physics of the specific phenomena, and I will provide introductory
information for most of them. However, all of these models are grounded
on a basic and unifying physical mechanism: the balance of capillary
and frictional (e.g. viscous) forces. Enlightening this principle will
hopefully help understanding and enjoying the subsequent lectures
within this series. |
2
Marzo
ore
12.00-14.00
Aula
Consiglio
|
Vittorio Loreto
Recent advances in language dynamics
 Language
dynamics is an emerging field that focuses on all processes related to
the emergence, evolution and extinction of languages. Recently the
study of the self-organization and evolution of language and meaning
has recently led to the idea that a community of language users can be
seen as a complex dynamical system that collectively solves the problem
of developing a shared communication framework through the
back-and-forth signaling between people. Language
dynamics is an emerging field that focuses on all processes related to
the emergence, evolution and extinction of languages. Recently the
study of the self-organization and evolution of language and meaning
has recently led to the idea that a community of language users can be
seen as a complex dynamical system that collectively solves the problem
of developing a shared communication framework through the
back-and-forth signaling between people.
In this talk I will review some of the progresses made in the last few
years and highlight potential future directions for the research in
this area. I will discuss in particular several examples corresponding
to the early stages of the emergence of a language, namely the
emergence of a common lexicon and the emergence of a shared set of
linguistics categories. I will point out how synthetic modeling has
nowadays reached sufficient maturity to contribute significantly to the
ongoing debate in cognitive science. For instance it has been recently
possible to reproduce in a numerical model the outcomes of an important
experimental survey, the so-called World Color Survey (WCS).
In addition new experimental frameworks are becoming progressively
available. Finally I will discuss the crucial issue in linguistics of
whether structures of languages we adopt are the outcome of an
individual-based process of optimization or rather the result of a
complex socially-driven cultural negotiation. I will argue that a
general scenario in language dynamics could be such that shared
linguistic conventions would not emerge as attractors, but rather as
metastable states.
|
3
Febbraio
ore
12.00-14.00
Aula
Consiglio
|
Steven
Strogatz
Social networks that balance themselves
Consider
a fully-connected social network of people, companies, or
countries, modeled as an undirected complete graph with real numbers on
its edges. Positive edges link friends; negative
edges link enemies. I'll discuss two simple
models of how the edge weights of such networks might
evolve over time, as they seek a balanced state in which "the enemy of
my enemy is my friend." The mathematical
techniques involve elementary ideas from linear
algebra, random graphs, statistical physics, and differential
equations. Some motivating examples from international relations and
social psychology will also be discussed. This
is joint work with Seth Marvel, Jon Kleinberg,
and Bobby Kleinberg.
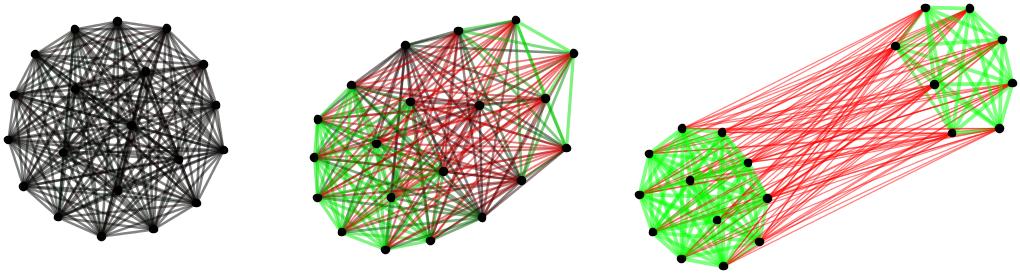
http://dueallamenouno.comunita.unita.it/2012/02/04/i-nemici-dei-miei-nemici/
|
13
gennaio
ore
12.00-14.00
Aula
Consiglio
|
Maurizio Battaglia
Modeling volcano deformation made easy:constraining the source of the
2004-2011 volcano unrest at Mount St Helens (WA).
Precise
measurements of ground deformation have become increasingly common as
large networks of GPS receivers and borehole strainmeters have been
established over the last decade. Complementing this continuous record
are comparatively infrequent but spatially dense images of ground
deformation from radar satellites, and a long historical record of
leveling, Electronic Distance Meter, triangulation, and tilt data.
Deformation can arise from tectonic and volcanic forces and from human
activities such as aquifer withdrawal or geothermal exploitation.
Mathematical models of how the crust deforms in response to different
physical processes are required to characterize driving processes and
constrain source location, size, orientation, and strength. This
information is valuable for hazards forecasting and mitigation.

|
|